I wrote a small piece on fair use of our biotechnology on my Tumblr, but since I’m planning to write longer pieces on this blog, I wanted to cross-post it here as well.

Joe Hanson, of It’s Okay To Be Smart, wrote:

Can Biotechnology and Genetic Engineering Save an American Icon?

What I find so interesting is that the techniques being used to save this tree, and one day reintroduce it to the wild, are not that different from those that are used to create genetically modified crops. How does saving a dying species by inserting a gene differ from creating an herbicide-resistant soybean, or rice that produces extra vitamins? I have my opinions, but I want to know: What do you think?

My thoughts are below.

The two are different in only the following way—in one case, we’re using our technology to help another being survive better; in another case we’re using technology to extract more from a being we intend to use as a ‘resource’, in this case food.

I think they’re both fine uses of our technology.

As humans, we have always wanted to modify our surroundings to suit us better. That’s who we are; that’s what defines us as a species and helps us move unflinchingly deeper into the unknown.

It was the same when we invented agriculture; it was the same when we domesticated animals; it was the same when we forced natural selection to go in a certain direction to create “man’s best friend”.

The only difference today is that instead of indirect approaches, we’re learning to make pin-point, particular modifications exactly as we require.

Yes, this is a sensitive topic, and rightfully so. With great power does come great responsibility, and we’re only now learning to harness the power of genetic engineering. I feel we should find it easy to stay on the straight and narrow as long as we remember one rule—no interference for the fun of it. I’ll explain more.

Only organisms capable of photosynthesis are able to produce their own energy. Every other living being must depend on other living beings for energy and sustenance, and we are no different. As long as our genetic engineering endeavours are focussed towards areas that we must harvest for our nutrition, we should be okay. Genetically modified crops are okay—as long as we understand the effects of what we are doing. Edit*: There is a lot of ambivalence towards genetically modified food crops, but the problem isn’t the technology itself, but that we don’t yet understand* the technology well enough to implement it perfectly. Let’s keep at it; we’ll get there.

In addition, being the sole species on this planet with advanced technology, we owe it to our planet-mates to share. Just as in this example of chestnut trees dying from a fungus, when we see an organism dying from infection, and we realize we can help—by all means, we should! We already try to help species that we are afraid will become extinct (often, unfortunately, to our own greedy exploits)–why should that help not include our latest and greatest knowledge?

Let’s just not play with our planet-mates simply because we can. That would be abuse of power, no?

I was lamenting on the scarcity of engineering blogs, even though there are a plethora of excellent science and other technical blogs on the internet.

That got me thinking about why relatively so few scientists in general, and engineers in particular, write and publish on the web. Here’s the problem, I think–

• We never receive any proper writing training throughout our careers.

  We learn the other stuff, all the theories and how they work and so on, and even how to publish our work in peer-reviewed journals, but rarely how to competently and forcefully express ourselves and communicate with the world at large. That’s a problem, isn’t it? After all, a scientist is as much a writer as anyone else—what use is my earth shattering research if I can’t explain it to everyone else?

  And no, ‘math does the talking’ is no excuse. Math isn’t for everyone, and it’s very useful to be able to communicate ideas outside of mathematical jargon. Even a brief “Here’s an idea. Now if you really want to know, go learn the math!” is extremely valuable.

  Here’s an example, via an article at Project Wordsworth: the Japanese mathematician Shinichi Mochizuki posted four papers on the internet, purporting to prove the ever-enigmatic ABC conjecture. The only problem? No one understands his work:

  The question which quickly bubbled to the top of the forum, encouraged by the community’s “upvotes,” was simple: “Can someone briefly explain the philosophy behind his work and comment on why it might be expected to shed light on questions like the ABC conjecture?” asked Andy Putman, assistant professor at Rice University. Or, in plainer words: I don’t get it. Does anyone?

  Oops! (And remember, we’re talking about the mathematics community here, not the lay public.) Dr. Mochizuki was invited to give lectures on his work, to explain and educate. He refused.

  Of course, his peers are irked:

  “You don’t get to say you’ve proved something if you haven’t explained it,” [former math professor Cathy O’Neil] says. “A proof is a social construct. If the community doesn’t understand it, you haven’t done your job.”

  If you can’t communicate, are you really a great researcher?

  Mochizuki has reported all this progress for years, but where is he going? This “inter-universal geometer,” this possible genius, may have found the key that would redefine number theory as we know it. He has, perhaps, charted a new path into the dark unknown of mathematics. But for now, his footsteps are untraceable. Wherever he is going, he seems to be travelling alone.

  This is, of course, an extreme case, but I think the larger point holds too—that engineers/scientists should be able to competently express themselves and communicate with the larger community, and not only in journal articles.

• We are not trained to be truly internet-savvy.

  I don’t mean this in terms of knowing how to navigate the internet and check email and visit websites and perform Google searches. I mean this in a larger sense—in knowing (and being comfortable with) how to create and maintain blogs, in managing our internet personas and profiles, in creating and designing websites.

  There are ample tools and resources out there, and we don’t all need to be trained in computer science to thrive—but we often rarely know how and where to begin. Some take the time to teach themselves, but what of those of us whose knack is not in internet technologies? We really do need to do more to expose ourselves more to internet publishing.

  We personally and professionally know of many scientists and researchers who are truly great teachers and communicators—but how many of these brilliant people are writing and publishing on the internet for the community at large?

If you’re an engineer or a scientist, and are a good communicator, please do consider writing and publishing on the internet! The rest of us will be the richer in experience for it. :)

I wish there were more people writing about engineering mechanics research. It’s certainly a fascinating area, and while perhaps they wouldn’t be as popular as the tech-media blogs, or the awesome science blogs that everyone can identify with, they’d still be pretty good, right?

I really like and follow Dr. Drang, who seems to occupy the perfect niche—mechanical engineering and computer programming. And through Dr. Drang I’ve recently discovered the blog of J. Ben Deaton, but haven’t had the chancce to explore in detail yet. (BTW, Deaton’s site is also powered by Octopress, with the default Octopress theme that I mentioned.) Then there’s Engineering is Awesome, which is also excellent.

But other than that, I don’t know of any engineering or mechanics blogs. There may be some great ones that don’t show up in Google searches—if you know of one, would you let me know? :)

There are quite a few science blogs though (example, example), and they are excellent and fascinating. But where are the engineers? Are engineers really that boring compared to other scientists? :)

I’d originally designed and built this website in the summer of 2011, and I had made it a point that I myself did all the hard yards of learning the technology and developing (and finding how others had implemented a feature) the HTML and CSS code. I stayed completely away from Javascript, mostly because I basically had no idea about Javascript. I wasn’t sure how resource-hogging Javascript was, and that was a factor, yes—but it was mostly because I didn’t want to use something I didn’t know, and I knew next to nothing about Javascript.

So that was then.

For a while now, though, I’ve been pondering a rewrite and rebuilding of the website, for a number of reasons.

• The website was not responsive to different screen sizes. It had a certain minimum width that it required, and it always served the same webpage. In today’s world of smartphones and tablets, while it was passable, it wasn’t elegant by any means.

• As written, it was a pain to make changes to the website. I was dealing with separate HTML pages, and making any changes meant going in manually and make changes to every single page. Tedious, and prone to error.

  I needed a way to make a change once and have it propagate throughout all my HTML pages.

• I needed to consolidate my writing options. Let me elaborate, and let’s see how convoluted it gets.

  I already have my wordpress blog–GlobeTrekker–which I like and want to continue to maintain—not least because I have certain Google pagerank on there that I don’t want to lose.

  I had started a new blog, a self hosted wordpress blog, on this website, that I had planned would be a “science and technology” blog, separate from ‘everything else’ that I write at GlobeTrekker. But this blog was not the face, so to say, of my website.

  In trying to think of what I could/should write on my “home” page, I decided that I’d use that space to write tutorials about the stuff that I know best about—composite materials, health monitoring, and eventually, even transportation research that I work on at VTTI. I was very excited with this prospect, and managed to write three—count’em, three!—posts, in two years. (There was a reason for this, which I’ll come to.)

  The problem was that I was increasingly finding it inconvenient to write on either of my wordpress blogs. (The reasons for that are for another post altogether.) So I started yet another blog, hosted at Tumblr, that I’ve been using much more productively in the past few months.

  As you can probably start to guess, it was getting tedious and completely inefficient.

• There was a problem with trying to write tutorial posts (mentioned above) on my home page. The problem was, every time I wrote a new post, there was quite some amount of work neeeded to just post that new material. The previous post had to find a new webpage all to itself. This page had to have links to earlier and later posts. The new homepage had to add new links to the new webpage just created.

  On top of that, every time I did this, Google’s search results went crazy. People searched on Google and found links to my home page, but of course that old content was now at a new webpage. Bad for the person searching, bad for my Google pagerank.

  Tedious, cumbersome, inefficient.

  See a pattern here?

–

So, in short—I needed some answers. And then recently, and finally, I stumbled upon Octopress.

In short, Octopress is a website building tool. It’s basically a Ruby program that does a number of things right out of the package.

• Assembles pieces of code to create the required webpages and support files to build an entire website. This straightaway solves my problem of having to make the same changes across all my pages. With Octopress, I make the changes in the one place where I need the change, and Octopress incorporates that change into all the web pages.

• Creates a blog, which is completely self-contained and self-hosted. Since it assembles everything locally, there’s no need for wordpress-style php pages on the server that handles collating pieces of content to serve a webpage.

  Octopress does it all, once, on my machine, and thereafter it’s just there, available for use.

• It provides a default theme of its own, but of course I am free to modify the code and designs as much or as little as I want.

  This is excellent, because now I can have all my web pages, including blog pages, with a consistent design. Getting this done with wordpress-hosted blogs is a pain, and while Tumblr is much better with this, it’s still infinitely more convenient to just have to do everything once.

  The website it creates is built to be responsive to different screen sizes. I’d been reading up on @media screen CSS usage, but having this built-in of course helped a lot.

–

So here we are, with Octopress. What you see now is the result of the redesign and the rebuild. Here’s what has happened:

• The front page now hosts a blog. This single blog will host the mechanics tutorials that I had mentioned (see here), and also host other pieces that I will hopefully (and am planning to) write.
• The Tumblr remains the best place to share content that I find interesting on the internet, and I will continue using that, but mostly for sharing with the odd comment. I will try not to write longer pieces on Tumblr. However, I’ll probably cross-post whatever I write on this website on Tumblr, just to reach a wider audience.
• GlobeTrekker, of course, remains—with the same mission statement. I don’t know how frequently I will write there, though.
• The self hosted blog will be deprecated. I haven’t removed the files themselves yet, but the posts there have almost all been mirrorred on the new website, and I will remove the old files over time.

So that’s it. New website, new technologies.

And I’ve become much more comfortable with including Javascript on my website since before, now that I’m more confident that I know the basic HTML and CSS technologies well. There are some excellent specialized Javascript tools (such as the brilliant Modernizr and jQuery), that I’d be foolish to not use—and which Octopress, of course, uses out-of-the-box.

How do you like it? There still are improvements to be done, of course—and more of Octopress’s built-in code to be dug into, but that’ll happen over time. I’ve disabled comments for now to make for a cleaner interface, so please use twitter or email to respond.

Welcome to my new website—and happy surfing! :)

Previously, we talked about what composite materials are, in an engineering sense. To recapitulate, composites are materials comprising two or more constituents. The constituents are combined in a way such that they retain their distinct identities in the final material (unlike alloys, for example). In particular, we talked about composites with a homogenous ‘matrix’ material (such as epoxy resin in polymer composites, and metals such as aluminum in metal matrix composites) in which reinforcing fibers (such as carbon fibers or glass fibers) or particulates are embedded. The fibers are the reinforcing material that provides strength to the composite, while the matrix material serves other purposes such as: (a) protecting the fibers (b) binding the fibers together to actually create the composite (c) helping to redistribute stresses if a fiber breaks.

But the key question is: why use composite materials at all? Why not use metals as always? What advantages do composites provide? Turns out, quite a few.

For one, composites are stronger than traditional industrial materials. How is strength measured? We all intuitively know this—by the amount of load that a material can withstand. Of course, for a fair comparison, the area over which the load (force, in technical parlance) is applied must be the same. (A thicker piece of wood carries more load than a thinner piece, but the wood itself remains the same strength; only the area of loading—the thickness, in this case—changes.)

A Mercedes-Benz bicycle that has a composite frame. (Source)

For another, most composites are lighter than their traditional counterparts. This is measured by density, which is the weight of the material per unit volume (just like we had considered constant area in the case of force, we must consider constant volume when considering weight). Like the example before, a larger piece of wood weighs more than a smaller piece, but the density of the wood itself remains the same.

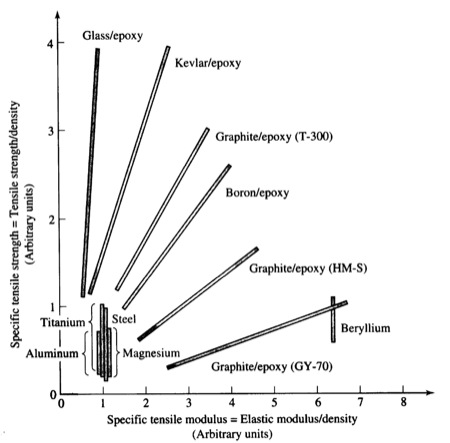
Comparison of material properties, normalized by density. (Longer is better. Notice the metallic materials at bottom left.) (Source)

Combine the two traits—lighter and stronger—and what we get is a material that can withstand the same load with a smaller amount of material, and the material itself weighs less for the same volume! This is a pretty neat arrangement, no?

It doesn’t end there; this is just the beginning.

–

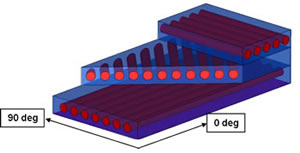
Remember, most of the strength of a composite material comes from the fibers in the composite. Now consider a composite with all the fibers parallel to each other, i.e. pointing in the same direction. In which direction (or directions) does the composite have the most strength? Is it equally strong in all directions? Evidently not—the fibers provide tensile strength along its own direction, and so the composite itself will be much stronger in the ‘fiber direction’ as compared to other directions. We’ve discussed wood as an example before, and you’ll notice that the situation is very similar to this case of unidirectionally arranged fiber-reinforced composites.

Load on a table top: notice the fiber direction! Here the requirements are bending strength for the table top, and ability to transfer the load to the legs of the table. (Source)

Now that we’re comfortable with the idea of a unidirectional composite, consider this material as a building block. If you had a unidirectional material, that you could orient any way you like, and stack them so that the final material had fibers in multiple orientations, could you design a material to be strong in any direction you wanted? Certainly you could! And this is exactly what is done.

Composite Ply Layup. (Source)

And this brings us to the engineering and design side of composites. Even though, at first look, this seems like a dubious idea—isn’t it better that the things we make are equally strong in all directions? What if it breaks in one of the weaker directions?–this makes good engineering sense. Whenever a new component is designed, the designer has already figured out what the weak points of the structure are, and has already planned a way (or ways) that the component should fail, should it become overloaded or reaches the end of its life. In other words, a good engineering design factors in, during the design process, the directions that the component needs to be strong in, and the ways the material can and should fail.

Of course, this implies that the components perform at their best when they are used as intended. If a golfer strikes his golf club in frustration against a tree trunk, should he be surprised if his prized club goes out of shape? (On the other hand, if many golfers do this exact same thing, the designer of the hi-end golf club might take this into consideration when he makes a new design—but it probably will make the club even more expensive!)

A bent golf club, after it was struck against the ground. (Source)

And of course, what of the case where the component does actually need to be equally strong in all directions? Well, there’s nothing preventing the designer from using plies oriented in all directions, right? A component where the fibers are oriented so that the properties are approximately the same in all directions is called a quasi-isotropic material, i.e. a material that behaves sort of like an isotropic material, even though it actually isn’t.

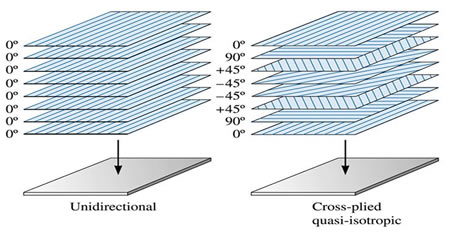
A unidirectional and a quasi-isotropic laminate. (Source)

One final thing for this session. How to describe the ply sequence of composites? In brief (I’ll clarify as we come across actual examples), the most basic nomenclature is just a sequence of the play angles, with one of the fiber directions designated the ‘zero degree’ direction. Since it can get cumbersome to write the sequences of multi-layer composites (consider a 16-ply or 32-ply laminate, for example!), symmetries and repetitions in the order of the plies is used profitably. The subscript ‘S’ denotes symmetry, ‘N’ (where N is a number) denotes N repetitions of the same order, and ‘T’ conveys that no symmetries and repetitions are present (i.e. it is the ‘total’ sequence). Please see the figure below for some examples.

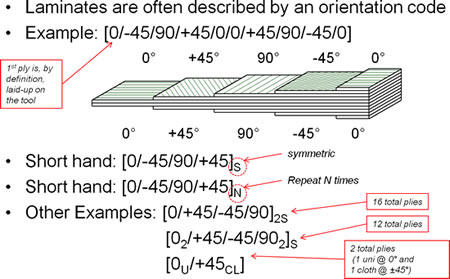
Composite stacking sequence nomenclature. (Source)

To summarize, the advantage of composites is first in its most basic properties—it is both stronger and lighter than traditional metallic materials. (It must be noted that this is a simple generalization; ‘strength’ can be of various kinds. For example, composites are usually not very good in compression). Further, composites can be engineered as per design requirements of each component. A basic unidirectional building block can be used to prepare composites with a variety of effective material properties.